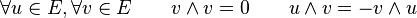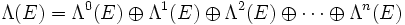Algèbre extérieure

Pour les articles homonymes, voir Algèbre (homonymie).

Cet article ou cette section concernant les mathématiques doit être recyclé.

Une réorganisation et une clarification du contenu est nécessaire. Discutez des points à améliorer en page de discussion.

En mathématiques, et plus précisément en algèbre et en analyse vectorielle, l'algèbre extérieure d'un espace vectoriel E est une algèbre graduée, notée $\Lambda^*E$ . Une présentation informelle de cette algèbre est donnée dans la première partie ci-dessous.

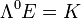Pour simplement comprendre les notations : * désigne ici la graduation. Elle est entière et commence par 0. L'ensemble $\Lambda^kE$ est un espace vectoriel sur le corps K. Ses éléments sont exactement ceux de degré k. Au moins en géométrie, ils sont souvent appelés des k-multivecteurs, ou plus simplement bivecteurs pour k = 2. En particulier, $\Lambda^0E=K$ et $\Lambda^1E=E$ . L'espace vectoriel $\Lambda^*E$ est la somme directe des $\Lambda^kE$ où k décrit N. Sa loi multiplicative, appelée le produit extérieur, est notée par le symbole wedge $\wedge$ . Elle est associative, bilinéaire et alternée. On dit qu'elle est "anti-commutative" ou "commutative au sens gradué" (voir plus bas).

L'algèbre extérieure est aussi appelée algèbre de Grassmann nommée ainsi en l'honneur de Hermann Grassmann. Vers 1840, ce dernier a écrit un traité sur les "grandeurs extensives", précurseurs des multivecteurs.

L'algèbre extérieure est particulièrement utile en topologie algébrique et surtout en géométrie différentielle et en physique mathématique. Elle permet en particulier de définir les formes différentielles et les champs de multivecteurs. En géométrie algébrique, l'algèbre extérieure intervient dans l'étude des faisceaux localement libres. Ces applications sont à peine abordées dans cet article qui se veut avant tout introductif.

Une première approche

L'algèbre extérieure peut être caractérisée comme l'algèbre la « plus simple » possédant les propriétés précédentes, ce qui s'exprime formellement à l'aide d'un problème universel. Dans un premier temps on peut se contenter d'une description par générateurs et relations.

Les éléments de la forme $v_1\wedge v_2\wedge\cdots\wedge v_k$ avec v₁, …, v_k dans E sont appelés k-vecteurs. Le sous-espace de Λ(E) engendré par tous les k-vecteurs porte le nom de k-ème puissance extérieure de E et se note Λ^k(E). Les éléments de cet espace sont donc des combinaisons de k-vecteurs, pas forcément des k-vecteurs.

L'algèbre extérieure apparaît comme la somme directe des puissances extérieures successives :

\Lambda(E) = \bigoplus_{k=0}^{\infty} \Lambda^k E

L'indice k forme un degré compatible avec le produit extérieur : le produit d'un k-vecteur et d'un l-vecteur est un vecteur de degré inférieur à k + l. Ainsi l'algèbre extérieure a une structure d'algèbre graduée.

Les relations

\forall u \in E, \forall v \in E \qquad v\wedge v = 0 \qquad u\wedge v = - v\wedge u

ne sont vraies en général que pour des vecteurs, pas pour des k-vecteurs, ni des éléments de l'algèbre extérieure.

Une interprétation géométrique des k-vecteurs : le 2-vecteur $u\wedge v$ représente le parallélogramme orienté de côtés u et v, le 3-vecteur $u\wedge v\wedge w$ représente le parallélépipède orienté de côtés u, v, et w.

Base et dimension

Si E est de dimension n et de base (e₁, ..., e_n), alors il est possible de donner une base de la k-ème puissance extérieure Λ^k(E), sous la forme

\{e_{i_1}\wedge e_{i_2}\wedge\cdots\wedge e_{i_k} \mid 1\le i_1 < i_2 < \cdots < i_k \le n\}

En effet, c'est un résultat général de décomposition pour les applications multilinéaires alternées. Chacune des composantes du k-vecteur sur cette base est un mineur de la matrice représentative du système de vecteurs v_j sur la base e_i.

La dimension de Λ^k(E) est le coefficient binomial ${n \choose k}$ . Notamment, Λ^k(E) = {0} pour k > n.

L'algèbre extérieure est une algèbre graduée égale à la somme directe

\Lambda(E) = \Lambda^0(E)\oplus \Lambda^1(E) \oplus \Lambda^2(E) \oplus \cdots \oplus \Lambda^n(E)

(dans laquelle Λ⁰(E) = K et Λ¹(E) = E), et sa dimension est donc 2ⁿ.

Définitions formelles

L'algèbre Λ*E est l'algèbre graduée la plus générale contenant E, avec un produit ayant la propriété d'alternance. Il est naturel de voir dans ce problème une variante de l'introduction de l'algèbre tensorielle T(E), et d'obtenir la propriété d'alternance par un quotient adapté. Soit donc I l'idéal bilatère de T(E) engendré par les éléments de la forme v⊗v pour v appartenant E (cet idéal contient les éléments de la forme v⊗w+w⊗v pour v et w appartenant à E). L'espace ΛE est défini comme le quotient ΛE=T(E)/I.

Portail de l’algèbre

This article is issued from Wikipédia - version of the Saturday, February 21, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.

Algèbre extérieure

Une première approche

Base et dimension

Définitions formelles

Medical Encyclopedia